At the base of the symmetric key algorithm figures, of those that use for encryption a simple, secret key, are the elementary figures the transposition and the substitution.[4]
The transposition figures realize a permutation of the characters of the cleartext. The encryption key is the pair K = (d, f), where d represents the length of the successive blocks of characters that will be encrypted according to the permutation f. The decryption is obtained by performing the inverse permutation.
Substitution cipher schemes replace each character in the message alphabet A with a character in the C cryptogram alphabet.
If 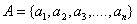then , where f substitution function, representing the key of the algorithm. Its form is: .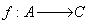
Traditional coding techniques are based on the sender's and recipient's knowledge of the encryption key. The sender encodes the message with a particular encoding system using the secret encryption key, and the recipient decodes that information using the same secret key. No other user needs to know the coding/decoding key.
There are two types of symmetrical encoding: stream-level encryption and block-level encryption. Bit-level encryption consists of encoding each bit of information, while at the block level a certain number of message bits are encoded simultaneously (for example 64 bits), called a block. Symmetrical encoding is faster than asymmetric encoding. A number of symmetric algorithms can be implemented in hardware. In this way, an algorithm becomes faster in operation.
There are two types of symmetric encryption algorithms:
1.      Block algorithms. Set lengths of bits are encrypted in blocks of electronic data with the use of a specific secret key. As the data is being encrypted, the system holds the data in its memory as it waits for complete blocks.
2.      Stream algorithms. Data is encrypted as it streams instead of being retained in the system’s memory.
Some examples of symmetric encryption algorithms include:
·         AES (Advanced Encryption Standard)
·         DES (Data Encryption Standard)
·         IDEA (International Data Encryption Algorithm)
·         Blowfish (Drop-in replacement for DES or IDEA)
·         RC4 (Rivest Cipher 4)
·         RC5 (Rivest Cipher 5)
·         RC6 (Rivest Cipher 6)
AES, DES, IDEA, Blowfish, RC5, and RC6 are block ciphers. RC4 is stream cipher.
The most commonly used symmetric algorithm is the Advanced Encryption Standard (AES), which was originally known as Rijndael. This is the standard set by the U.S. National Institute of Standards and Technology in 2001 for the encryption of electronic data announced in U.S. FIPS PUB 197[1]. This standard supersedes DES, which had been in use since 1977. Under NIST, the AES cipher has a block size of 128 bits, but can have three different key lengths as shown with AES-128, AES-192 and AES-256.
 
Symmetrical cryptography also has some disadvantages, such as:
-          Does not ensure the authentication of the sender. This security gap does not allow the electronic verification of certain transactions;
-          The transmission of the secret key between correspondents must be carried out on very secure channels.
-          When used between network users, a large number of secret keys are required to communicate between two users.
AES
One of the more popular and widely adopted symmetric encryption algorithms likely to be encountered nowadays is the Advanced Encryption Standard (AES). It is found at least six times faster than triple DES.
A replacement for DES was needed as its key size was too small. With increasing computing power, it was considered vulnerable against exhaustive key search attacks. Triple DES was designed to overcome this drawback but it was found slow.
The features of AES are:
·         Symmetric key symmetric block cipher
·         128-bit data, 128/192/256-bit keys
·         Stronger and faster than Triple-DES
·         Provide full specification and design details
·         Software implementable in C and Java
 
AES is an iterative scheme. It is based on ‘substitution–permutation network’. It comprises a series of linked operations, some of which involve replacing inputs with specific outputs (substitutions) and others involve shuffling bits around (permutations).
AES performs all its computations on bytes rather than bits. Hence, AES treats the 128 bits of a plaintext block as 16 bytes. These 16 bytes are arranged in four columns and four rows for processing as a matrix −
The number of rounds in AES are variable and depend on the length of the key. AES uses 10 rounds for 128-bit keys, 12 rounds for 192-bit keys, and 14 rounds for 256-bit keys. Each of these rounds uses a different 128-bit round key, which is calculated from the original AES key.
The schematic of AES structure is illustrated in figure 2:

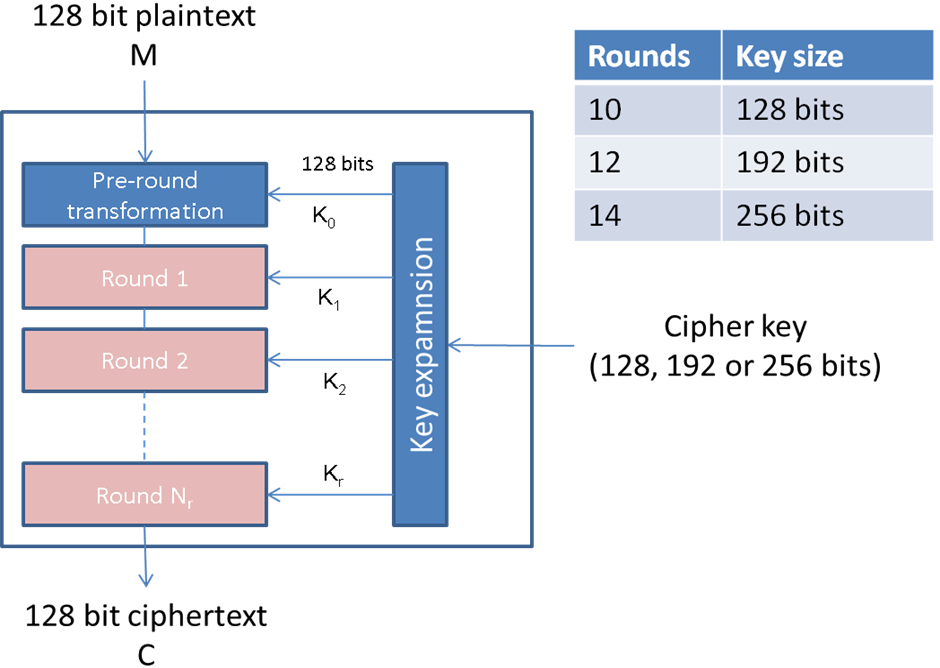
Figure 2. AES scheme

Encryption Process
Here, we restrict to description of a typical round of AES encryption. Each round comprise four sub-processes, as follows: AddRoundKey, SubBytes, ShiftRows, and MixColumns[1].
  
Pseudo Code for the AES cipher is:
Cipher(byte in[4*Nb], byte out[4*Nb], word w[Nb*(Nr+1)])

where:
Byte Substitution (SubBytes)
The 16 input bytes are substituted by looking up a fixed table (S-box) given in design. The result is in a matrix of four rows and four columns.
Shiftrows
Each of the four rows of the matrix is shifted to the left. Any entries that ‘fall off’ are re-inserted on the right side of the row. The shift is carried out as follows:

• The first row is not shifted.
• Second row is shifted one (byte) position to the left.
• The third row is shifted two positions to the left.
• The fourth row is shifted three positions to the left.
• The result is a new matrix consisting of the same 16 bytes but shifted with respect to each other.

MixColumns

Addroundkey

Decryption Process

• Add round key

• Mix columns

• Shift rows

• Byte substitution

Since sub-processes in each round are in a reverse manner,  the encryption and decryption algorithms need to be separately implemented, although they are very closely related, as follow:
InvCipher(byte in[4*Nb], byte out[4*Nb], word w[Nb*(Nr+1)])

 
In present-day cryptography, AES is widely adopted and supported in both hardware and software. Additionally, AES has built-in flexibility of key length, which allows a degree of ‘future-proofing against progress in the ability to perform exhaustive key searches. However, just as for DES, the AES security is assured only if it is correctly implemented and good key management is employed.
AES has three different key lengths. The main difference is the number of rounds that the data goes through in the encryption process, 10, 12, and 14 respectively. In essence, 192-bit and 256-bit provide a greater security margin than 128-bit.
In the current technological landscape, 128-bit AES is enough for most practical purposes. Highly sensitive data handled by those with an extreme threat level should probably be processed with either 192 or 256-bit AES.

---

[1] https://nvlpubs.nist.gov/nistpubs/FIPS/NIST.FIPS.197.pdf